Close to Fifty! Developing Base Ten Concepts and Place Value with First Graders

Before you read on, imagine how a first grader might respond when asked to solve 23 + 20. Some might just stare at you, not having an entry point. Some might start counting from one, but soon get lost without the help of concrete materials like cubes. Others might start with 23 then count on 20 more by ones. And there are a few who might say, “Well, twenty and twenty is forty, plus three more makes forty three.” This last strategy, a powerful one that is efficient and often accurate, is based on place value and the ability to take apart the number 23. It’s a strategy that we want all first graders to eventually have access to. But how do they get there? 

 

The Foundations of Arithmetic

Nicholas Branca, former professor of mathematics at San Diego State University once told me that the foundations of arithmetic are counting, place value, and number decomposition. This makes sense because we know that a solid understanding of basic counting concepts helps children understand place value, and understanding place value allows students to break numbers apart and put them back together. 

Recently, Math Transformations consultant Olivia O’Donnell and I worked with a group of first grade teachers and their students in Vista, California exploring the game Close to Fifty!  In this post, we’ll take you into the classroom and share how these first graders engaged with the game as they practiced making sense of base ten concepts and place value at their own level of development.  But first, a few thoughts about the path children take from counting to place value. 

 

The Path from Counting to Place Value

In our post titled, Teaching Teen Numbers in Kindergarten, we focused on ways to build the foundation for place value in kindergarten. For those young students, building this foundation starts with developing fluency with counting and all that it encompasses, as well as making sense of teen numbers. 

Through experience, students move from counting ones to being able to unitize, a fancy word for a child’s ability to see numbers in groups, rather than always seeing numbers as individual units. So, for a child to look at the number 16 and really understand what it means, they should know that the ‘1’ is a group of ten and the ‘6’ means six more ones. Learning to unitize isn’t easy and takes lots of practice with rich counting and grouping experiences.

As students move from kindergarten to first grade, they still need these rich counting experiences working with ten-frames, double ten-frames, and larger frames like the fifty-frame we’ll be focusing on in this post. According to the Common Core State Standards for first grade, students are expected to “understand that the two digits in a two-digit number represent amounts of tens and ones.” While this is no easy task, the game Close to Fifty goes a long way in supporting first graders with this standard. 

 

Close to Fifty!

When we visited, the first-grade teachers worked in small groups with their students. I joined one group while Olivia settled in with another group. 

In my group, the teacher handed each student a Close to Fifty  gameboard (directions to the game and the gameboard are found using the link).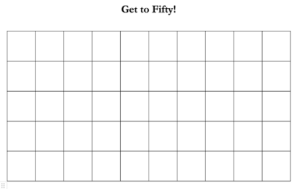

Before giving directions, the teacher asked her students what they noticed and wondered about the board. This was a smart move since it provided for an assessment opportunity. What would they notice? Would they estimate how many squares are on the board? Would they use what they know about ten frames to guide their thinking? Would they count by ones? Tens? Would students even notice that there are ten squares in each row? 

As you would expect, students were all over the map in terms of what they noticed and how they figured the total number of squares in a row and on the board.  Their comments ranged from noticing that ‘there are lots of squares’ to commenting that ‘there are five rows with ten in each row.’  This open-ended initial question offered the opportunity not only to assess students but also to establish with them that there are in fact ten squares in each row. Along with their teacher, the students counted the squares in the first row to double-check that there were ten. 

 

Playing the Game

After each first grader had a gameboard, a pair of dice, a pencil, and access to Unifix cubes, the teacher explained that the goal of the game is to cover all the squares with cubes to get close to fifty without going over.  Students would roll two dice, add the numbers together, and place that many cubes on their gameboard. As teachers, our job was to observe, ask questions, and clarify for students any questions they had about the game directions. 

These are some of the questions we asked:

To support seeing seeing a total quantity within the structure of the grid:

        “How many cubes do you have?  How do you know?”

To support seeing the distance to the next ten or multiple of ten:

        “How far are you away from _____?  How do you know?”

To support place value:

        “How many cubes do you have?  How many groups of ten cubes do you have?  How many extras?”

As we watched, we noticed lots of learning. The two boys pictured below worked together on the same gameboard and took turns rolling the dice. They rolled a 4 and a 5 and counted on from 5 to find the sum of 9. Other students used number sense strategies to figure sums. For example, one student when adding 5 and 6 doubled the 5 to make a 10 and then added one more to get to 11. Others counted all the dots on the dice to find sums.

 

Asking Counting Questions

When we asked Victor, in the photo above, how many cubes he had on his gameboard, he counted by tens, pointing to each row. “Ten, twenty, thirty,” he counted, and then stopped. He thought for a few seconds, then continued counting, “thirty-one, thirty-two, thirty-three, thirty-four, thirty-five.” Counting the extra ones after counting groups of ten can be challenging, but for Victor, the task was just right for him. 

Some students, when asked how many cubes they had on their board, always counted by ones. Even though students will often stick to their comfortable counting strategies, it doesn’t mean we can’t nudge them to consider counting groups with questions like, “Is there another way to count the cubes?” Or “Tell me again how many cubes are in each row.” We might even model for them counting rows of ten and extra ones, keeping in mind that showing or telling them won’t magically result in understanding. 

 

Asking Place Value Questions

When Victor and his partner reached 41 cubes (see below), I asked them how far away from 50 they were. Victor, rather than counting by ones the rest of the way, responded, “We’re nine away ‘cause the row has ten squares and there’s only one cube on it. Ten take away one is nine” 

I like this question because it’s a form of subtraction: how far away from 50 is 41? In other words, what is the distance (or difference) between the two numbers?

After the players figured that they had 41 cubes on their board, I shifted my question to focus on place value. I asked Victor to write the number 41 on the gameboard (see above). Then I asked, pointing to the ‘4’ in 41, “Show me what the four means on your gameboard.” Victor was able to point to the four groups of 10 cubes. Then pointing to the extra cube, he added, “And this is the one (in 41).” 

Understanding the meaning of the digits in a number is key, and it’s a developmental milestone in understanding place value. Knowing the place value of the digits in a number is different from a digit’s face value. On its face, the four is just a four. But in our base ten number system, where the four is placed in a number gives it its value. This is a tricky concept for young students. Games like Close to Fifty, and questions that prompt students to connect the digits in a written number to quantities of cubes arranged in groups of ten can really help with place value development. 

 

Differentiating Instruction

As students played Close to Fifty, some didn’t seem ready for a 5 x 10 grid. So, we switched gears and gave one pair of players a Close to Twenty game board (see below). Rather than roll two dice, a player rolls one die and places the cubes on the gameboard with the goal of getting close to 20. It’s essentially the same game but differentiated for students who aren’t yet ready to tackle 50. 

Concept of Ten: Developmental Levels

In their book Teaching Number (Corwin Press, 2006), Jim Martland, et. al, introduce a three-level model for the development of base-ten concepts that can be very useful when assessing your students (see below). As you read about the levels, try to visualize your own students and where they might fit in this model, keeping in mind that they often move forward and back between the levels as they learn and gain experience with counting tens and ones. 

 

We have found that the kinds of experiences  students have with counting groups (like Close to Fifty) and the types of questions  we ask them as they make sense of quantities make a big difference in their ability to move through these levels. We have also found that rushing children through these stages is not helpful to their learning. As early childhood expert Kathy Richardson once said, “When we push students too quickly through the curriculum, they just do the wrong things sooner.” 

 

Close to Fifty Addresses the Common Core Standards

The Common Core State Standards expect first graders to “understand that the two digits in a two-digit number represent amounts of tens and ones.” But the standards go further by expecting first graders to “add two-digit numbers and a one-digit number (23 + 5) and a two-digit number and a multiple of 10 (23 + 20), using concrete models (like Unifix cubes) or drawings and strategies based on place value (e.g. 23 + 20 = 20 + 20 + 3). Close to Fifty helps students with both standards, as well as practicing their basic addition facts. 

We hope you try out this game with your students, whether they are first or second graders. Even some third graders will benefit if they are still developing base ten and place value concepts. 

Have fun, and thanks Olivia and Vista teachers for your expertise and Vista first graders for your engagement and enthusiasm!