Helping Students Make Sense of Subtraction

The other day I was reading an opinion piece about teaching subtraction, and although I didn’t agree with some of the ideas in the article, it really made me think, talk to colleagues and friends, read what others have written about subtraction, and go in and try stuff out with kids. The author was writing about helping her 8-year-old son with his math homework. The problem he had to solve was 70-6.

The author was mystified by how the teacher (and the textbook) wanted students to solve the problem. She couldn’t figure out what she described as the “new way” of doing subtraction, and just wanted to explain to her son the “old way,” the way she learned it, which was the traditional algorithm of “borrowing” from the ten’s place.

 

 

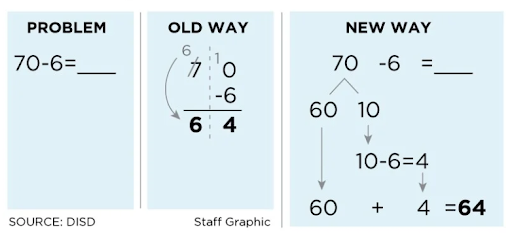
I thought about this for a minute or two, until I realized that the “new way” was really the same as the “old way” except that it clearly shows how you decompose the 70 into a 60 and a 10 before subtracting the 6, thereby reinforcing to students the idea of place value and number decomposition, two foundational ideas when learning arithmetic.

The standard algorithm was what the author was used to, it’s what she was familiar and comfortable with. And for many parents, “new ways” of doing math, even if they make more sense to children, can be threatening, especially if they are attached to what they know and understand. To the author, just explaining the “old way” seemed easier and faster. But explaining the standard algorithm for addition to a second grader can be confusing for them, and although it’s a short cut, it can also mask place value understanding.

How many times have we heard kids say things like, “cross out the seven (which is really a seventy) and put a six (which is really a sixty) next to it, then put a little one next to the zero to make it a ten, and then take six away from the ten.” Kids learn the script, but do they really understand what they are doing? In fact, the “new way” that the author talks about can be just as confusing to students if it’s taught as a procedure to be memorized rather than understood. As Albert Einstein once said, “Any fool can know. The point is to understand.”

So, what’s a teacher to do? Following are some ideas that can help students build understanding and gain fluency with subtraction.

Build Counting and Addition Fluency

When posed with problems like 70-6 or 70-68, many second graders would choose to just count forward or back by ones to get the answer. This seems like the most efficient strategy, given the numbers in the problem. Part of being procedurally fluent is knowing what strategies to use for the problem at hand. And knowing how to count forward and backward is an important skill for children to use for some subtraction problems.

Addition is much easier for young students than subtraction. And when solving subtraction situations, children will often use addition to get an answer. For example, when solving a problem like 85-70, many students will start at 70 and add up to 85 to find the answer (70+10 is 80; 80 plus 5 is 85; so, the answer is 15).

I was recently reading Marilyn Burns Blog in which she writes about a Number Talk she facilitated, where students solve problems mentally. She posed the following missing number problem, 90 – ___ = 75. To solve, many students either added up in chunks (75+5=80; 80+10=90; 5+10=15) or subtracted back from 90 in chunks (90-5=85; 85-10=75; 5+10=15).

Using the relationship between addition and subtraction, counting by 5s and 10s, and being able to add or subtract 5 and 10 from any number can all be powerful and useful skills while solving subtraction problems.

Offer Games that Provide Visual Models

Subtraction gets easier when students can ‘see’ the actions of subtraction when they use concrete or visual models.

One of my current favorite subtraction games is called Sticker Compare from Investigations. Use the link for Sticker Compare and then scroll down until you see the game. There is a sticker card deck for playing and full directions for the game here.

In the game, players each turn over one card from a deck of sticker cards and compare the quantities. For example, each player must ‘read’ the quantities on the two sticker cards below (37 and 47) and figure out who has more and how much more. The visuals support place value development and help students figure the answer to a subtraction ‘compare’ problem.

Use Number Talks

One way to help students develop understanding of subtraction is to pose problems during a Number Talk (see our posts about Number Talks). Pose a problem (like 70-6) and ask students to think of a way (or two) of solving it. Then have them share their strategy with a partner. Finally, elicit a few ideas from the class and record their thinking on the board to make their strategies visible. Chances are students will come up with various methods. And it’s a good way to introduce different ways of thinking about subtraction to the class. When my friend Lila posed the problem to her second graders, many counted back from 70 by ones and got the answer quickly and efficiently.

Pose a Variety of Subtraction Contexts

In a terrific blog post by Kristin Gray, she says that one reason students struggle with subtraction is because of the lack of variety in contexts that we present them with. In her work with second graders, she presented them with two different subtraction contexts to see how they would solve the problems. Here’s the first one, which Kristin calls a “distance context” (the distance between what Mike has saved and how much the game cost):

Mike saved 8 dollars to buy a game.

The game cost 22 dollars.

How much more money does Mike need?

Kristin noticed that every single second grader added up to solve the problem. Next, she posed this “take away” problem:

Bobby had 22 toy cars in his book bag.

He gave 8 of his toy cars to his friend Becky.

How many cars did Bobbie have left?

Every student except for one ‘took away’ to solve the problem. When they were finished, Kristin had the students discuss the two problems. She reported that “they noticed they were the same numbers and same answer, but one student exclaimed, “How can they be the same answer when we added in one and subtracted in the other?!” This comment, Kristin noted, was a great jumping off point for future lessons on subtraction.

Posing a variety of subtraction contexts can help students understand the actions of the operation and help them think about strategies to use when solving different subtraction contexts.

Highlight Using Friendly Numbers

Using friendly numbers can help students bypass having to decompose numbers thereby making problems easier to solve. For example, let’s look at the problem 63-37. Finding the closest friendly number for the subtrahend (changing 37 to a friendly 40 by adding 3) and then adding 3 to the minuend (63) to make 66, results in an easier subtraction problem to solve.

63 + 3 = 66
-37 + 3 = 40
26

Rounding to friendly numbers in compensation strategies can also be useful. In the problem 54-29 we can round 29 to 30 and make the problem easier if we remember to add back the one at the end. These strategies are helpful to students if they understand and make sense of them and can apply the strategies when appropriate.

Introduce Number Lines

When students use a number line, they can visually see the jumps they make, forward and back. They can hop by ones or by tens and see how addition and subtraction operates. When solving 9-7 for example, children can hop forward from 7 or back from 9 to land on the result.

For larger subtraction problems such as 144-82, students can solve the problem using different jumps on the number line. Following is an example of a student who used addition by adding up, then combined 18 and 44 to get the answer.

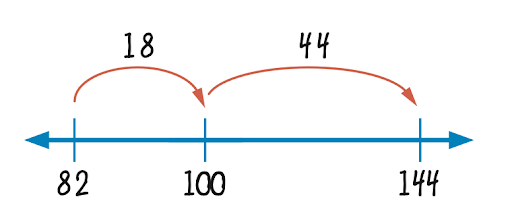
This student subtracted back from 144 and then added 8+ 10 + 40 + 4 to get the answer.

Back to the Article

One thing the author and I agree on is her argument about problems involving larger numbers. She challenges the reader by saying, “Try breaking 1,256-739 into component parts!” She’s right. When subtraction problems get bigger, using decomposing strategies can be unwieldy, and using algorithms that are more efficient makes sense, if they make sense to students.

The good news is that the Common Core Math Standards recommends delaying teaching the standard subtraction algorithm until fourth grade, thereby giving children more time in the early grades to build understanding of place value and properties of operations. By fourth grade, students are ready to use shortcuts like the standard algorithm when tackling bigger problems.

We hope this post gives you some ideas to help your students experience success with subtraction. For more ideas, check out this post by Jeanne Sager.