Through the Decimal Maze with Fifth Graders!

In a recent blog post (see Developing Decimal Number Sense), we talked about decimal number sense, what it is and how to develop it in our students. Defining decimal number sense isn’t easy, but it becomes clearer when we observe students using their number sense when solving problems. 

Students with decimal number sense understand what numbers mean, and they have a sense of the magnitude of decimal numbers. These students understand number relationships, and they can estimate the answers to problems because they have a good intuition about what will happen when we add, subtract, multiply, and divide decimal numbers. The game Decimal Maze, the focus of this post, helps students develop this intuition. 

 

Returning to Kathy Seckington’s Class

I had decimal number sense on my mind when I returned to Kathy’s fifth grade class to share Decimal Maze (Illuminations, NCTM 2008). I’d been to Kathy’s class earlier in the year (see Finding Success Teaching Math Word Problems), and I was excited to work with her students again and get a chance to co-teach with one of my favorite teachers.

Kathy’s students had been studying decimal numbers for a few weeks, reviewing the meaning of these numbers, using them with the four operations, and learning about the relationship between decimals and fractions. Kathy was curious to see what strategies her students might use during the game. Observing them play would be a good opportunity to assess their number sense.  

Before I visited, Kathy made sure that her students had prior experience using calculators, a key tool they would use when playing Decimal Maze.

 

Modeling the Game

Following are the directions for Decimal Maze that we shared with the class: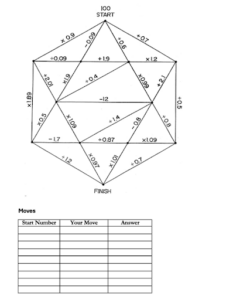

1. Begin with a value of 100 on your calculator. When you choose a segment to cross, you must estimate the result. Then cross the segment, perform the indicated operation on your calculator, and record the equation at the bottom of the game board. If you end up with a repeating decimal, round to the nearest hundredths or whole number. 
2. Move down or sideways (never up) through the maze from start to finish. You may not retrace your steps.
3. The goal is to choose a path that results in the largest value when you reach the finish line.
   

Kathy started by putting a copy of the Decimal Game Board and a calculator on her document camera for all to see. She placed a centimeter cube on the START position, entered 100 in the calculator, then Kathy and I began modeling a few moves for the class. 

As we modeled, we made sure to emphasize the importance of estimating the answer when choosing a pathway. For our first move for example, I turned to Kathy and said, “I think we should add seven tenths to 100,” modeling how to read a decimal number. “Kathy, what do you estimate the answer to be?” 

Kathy thought for a few seconds, then said, “I estimate the answer will be a little more than one-hundred, ‘cause we are adding a number less than one to one hundred. Let’s find out!” We used the calculator on the doc cam to compute and then showed the class how to record on the gameboard. 

Kathy and I modeled another move, chatting first about our estimate using the following frame which we posted on the board:

      I estimate that the answer will be _____ because _____________.

Here are our first moves:

Finally, we told the class that they would play the first game together with a partner using one cube to move, supporting one another rather than competing.

 

The First Game

Once pairs had their gameboard, calculator, and centimeter cube, they settled into their first game. Kathy and I circulated, listening in as players chatted about their pathway choices and estimates. We noticed lots of surprises!

For example, Jose and Jeffrey entered 100 ÷ 0.6. When they checked their answer, they gasped, “Wait, what?!” I asked them what they were surprised about, and they both couldn’t believe the answer was 166.66666.

“What was your estimate?” I asked. 

“A lot less.” 

“Why?” I probed. In unison, they both replied, “Because when you divide the answer should get smaller!” 

I suggested they try again on the calculator, and they were stumped when they arrived at the same answer. Jeffrey turned to me and said with a shrug, “I guess you can’t question a calculator!” 

Many students were surprised to find that when they divided by a decimal number, the answer got bigger, not smaller. The same with multiplication. Many thought the answer would always be bigger than the multiplier. Others were shocked by how big their total was when they got to the finish line. All in all, players were engaged, excited, and curious. 

 

A Class Discussion

Once students were finished with the game, I pulled them back together for a class discussion, prompting them with the following questions:

“What did you notice during the game? 

“What did you notice about the equations you wrote?”

“What are you wondering about? Surprised by?”

Most students noticed and were surprised by what happened when they divided whole numbers with decimals numbers (less than one or mixed decimal numbers), and how multiplication didn’t behave like it does with whole numbers. This is really what we wanted students to see because part of having decimal number sense is possessing an intuition about what will happen to numbers when we operate on them. 

Students spent a few minutes gathering their thoughts and writing them down. Here are a few examples. 

Alan wrote,” I was surprised by how high the number could get from 100. Will and I got more than 2000! I also saw dividing a whole number by a decimal made the number huge like when 90 ÷ 0.04 got us more than 1,000.”

Eva: “The more you divide, the bigger the answer.”

Sam: “I noticed that me and my partner had some struggles, and we had a hard time understanding the math, but it started to get easier as we went on and got the hang of it.”

Jill: “I noticed that dividing a number by 0.5 is like multiplying the number by two.”

Marco: “There were many choices on what to decide on. And I would have to choose wisely. Plan all moves beforehand!”

 

The Second Game: Using Strategies

When students were finished writing, Kathy had them find a different partner and play another game, this time it would be one player vs. another. For game two, each player needed their own new game board, a centimeter cube, and their paper for writing reflections.

Kathy had a great idea to have partners share one calculator; that way they wouldn’t end up playing by themselves and they would be more likely to talk with their partner about their learning as they played. 

Kathy urged the class to use what they learned from their first game to inform their strategies for getting the most points in the second game. 

As partners played against one another, Kathy and I circulated. We noticed that during the second game students were better able to use their calculators and write their equations. Some were so intrigued with using a strategy that they paused during the game to plan out a path that would yield the highest score. 

Everyone seemed engaged as they tested out strategies, motivated to get more points than their partner. Most players caught on that choosing a path that allowed them to divide was the best strategy. 

During game two, I noticed that some players were a little confused when trying to read their decimal numbers. For example, when looking at the number 166.6666, some students thought it was a really big number (they weren’t paying attention to the decimal point and just looking at the number of digits.) I had the class pause, and I wrote the number on the board and asked a student to read the number aloud. We had a nice discussion about how to read a decimal number, as well as when to spot a repeating decimal. 

 

Writing About Strategies 

When students were finished with game two, we had them write about the strategies they used and any final things that they noticed and wondered. Following are a few examples.

 

Nathan was surprised that multiplying a mixed number by a decimal number less than one resulted in a product that was smaller than the multiplier (see above).

 

Estella decided to change strategies and divide rather than multiply (see above).

 

Jason seems to be onto something when he refers to division as ‘groups of’ as in ‘how many groups of 0.5 are in 456 (see above). 

 

A Final Class Discussion

To wrap up the lesson, Kathy assembled the class on the front rug and facilitated a discussion. Her goal was for students to review what they’d learned during Decimal Maze. She started by asking them what they liked about the game. They had a lot to say.

Alexandria started. “I liked that I got to work on my estimating skills.”

Rather than just moving on to the next student, Kathy probed a little. “How does estimation help you?”

Alexandria thought for a few seconds, then said, “It helps because it can tell me if my final answer is really off or not.”

Mel jumped in, adding, “I liked the game because we got better with practice.” Kathy and I noticed this as well. 

“I liked playing with a partner and I learned a lot about decimals,” Abbie said. Others nodded in agreement. “I also liked that you had a lot of choices, different pathways to get points.”

 

Noticing and Wondering

Kathy then shifted her questioning by asking what students noticed and wondered while playing. 

“I wonder how big of a score you can get,” Evangeline said. “Some players got over 3000!”

“Nod your head if you got a really big score,” said Kathy. Lots of nods all around. “I wonder how that happened?”

“It’s when you divide the decimal numbers,” Leah noted. “It’s like what we discovered during the game. When you divide it gets bigger, the answer gets bigger.”

It was at that point that Kathy wisely asked the WHY question. “Why does the answer get bigger when we divide a whole number by a number less than one?”

There were a few great responses. For example, Ken said that it’s like 100 divided by 0.5, that’s like how many halves are there in 100. A lot!” Another student noted that 450 divided by 0.2 is like asking, “How many 2/10 are there in 450?”

While these were terrific examples, there wasn’t enough time at the end of the discussion for a deeper dive, but it did serve as a nice endpoint for one lesson and a rich beginning for another. There was so much more math to uncover, explore, and make sense of. 

 

Developing Decimal Number Sense

We found that having students play Decimal Maze was an amazing (pardon the pun) way to help them develop their decimal sense. The game engaged players in estimating, making decisions, selecting and trying out strategies, fine tuning their use of a calculator, and practicing the four operations with decimal numbers. Most importantly, the game helped Kathy’s fifth graders develop their operation sense as they gained insight into what happens when you operate on decimal numbers.

Thank you to Kathy’s fifth graders for their enthusiasm and brilliance! And thank you Kathy for allowing me to learn from you and for welcoming me into your classroom.